
Prob. Set 7 due November 9
Reading: Ch.6, p. 178 - 196.
Exam II: Given out Thur, Nov. 11, due 10am Tues, Nov.16. Covers through
Ch. 5.
Problems:
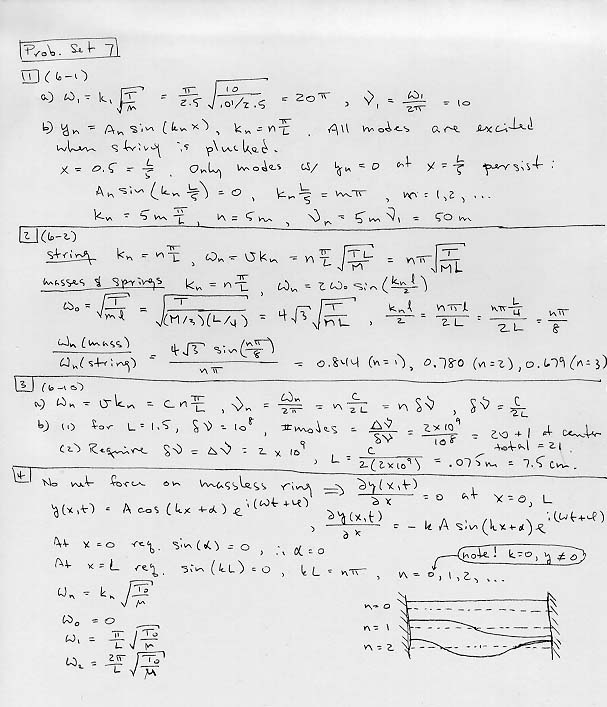
1) 6-1
2) 6-2
3) 6-10
4) Find the mode configurations and frequencies for the first three modes of transverse vibration of a continuous string with tension To, mass density m, and length L given the boundary conditions that both ends are free, i.e. they slide on frictionless rods that pass through massless rings at each end of the string. (Hint: what net force can be applied to a massless ring?)
5) The wave machine we used in class consists of a steel wire backbone along the x direction with transverse iron rods. Let k denote the angular spring constant of the wire, i.e. the return torque is k times the angle of twist (in radians). Let I be the moment of inertia of one rod. Find the dispersion relation, i.e. the relation between w and k, for the wave machine.
6) Here are some data for the wave machine:
The rods are 45.7 cm long and 0.40 cm in diameter.
The length of the machine from the first rod to the last rod is 90 cm.
With a 50 gm mass hung 15.3 cm from the wire backbone on rod #1, rod #6 must be twisted by 23.5° to keep rod #1 horizontal.
With the end rods clamped the period measured in class for the n=1 mode was 2.9s, for the n = 2 mode 1.7 s.

